This is Part 1 of a two-part article on process capability. Part 1 addresses the concept of process capability and how to calculate it, including what to do with data that is normal and non-normal. Part 2 looks at failure rates and material conditions.

What is process capability? From a conceptual view it is a measure of the relationship between the voice of the process and the voice of customer (VOC). It is essentially a ratio of the customer requirement (specification) and the expected process variation.

Process capability = Voice of the customer / Voice of the process

It is an expression of how well your process performs relative to the VOC. And it is a prediction of how well your process will meet customer requirements in the future. A capable process is one in which almost all measurements of a feature produced by the process fall inside specification limits. There are several indices that are commonly used.

Cp

Let’s use a car and garage example to drive home the concept of Cp. The garage defines the specification limits. The car size represents the process limits.

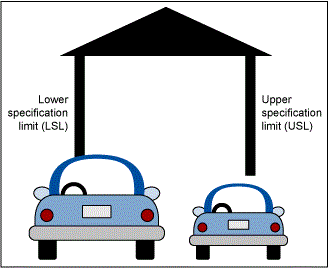
Figure 1: Cp
Figure 1: Cp

If the car is smaller than the garage, it means Cp > 1; the car will fit inside the garage. When you find that your data (car) is smaller than the specification limits (garage), your process is capable. It is, therefore, safe to conclude that you will not have problems meeting the specifications. In other words, you will not have problems parking the car in the garage. Cp is the specification width divided by the process width.

process capability

Cpk

In the Figure 1 illustration above Cp > 1, which as we just learned means the car should fit in the garage. . .if the car is always centered.

Figure 2: Cpk
Figure 2: Cpk

However, when the car isn’t centered, you are at risk of damaging the car as well as the garage – or, in process terms, falling outside of the specification limits and not meeting customer requirements. Another process measure is needed to address the centering of the car in the garage. Cpk to the rescue! Cpk tells how much clearance can be expected from the side of the car to the nearest edge of the garage. Look at the distance from the center of the car to the nearest edge of the garage, then divide that by half of the width of the car.

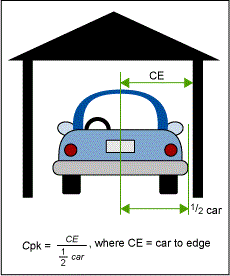
Figure 3: Customer Expectation
Figure 3: Customer Expectation

Consider CE as customer expectation. The customer expects the car to fit inside the garage and that the car will be reasonably centered in the garage. The customer of your process has similar expectations. For a process:

Process Capability

USL = upper specification limit
LSL = lower specification limit
 = estimate of the process’s standard deviation

Conceptually, the standard deviation is the average spread of the data about the mean.

Quality guru Dr. Walter Shewhart taught that a process is behaving normally when it varies by no more than ±3. (for a total spread of 6σ). Therefore, the denominator of the Cpk calculation is 3 (6σ divided by 2). Cp is always a positive number as it is the ratio of two positive numbers. Cpk can be positive, zero, or negative.

Cp and Cpk

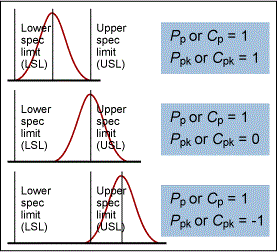
The relationship between Cp and Cpk is shown in Figure 4.

Figure 4: Relationship Between Cp and Cpk
Figure 4: Relationship Between Cp and Cpk

Process Capability Assumptions

When calculating process capability Cp or Cpk, there are three key assumptions:

  • Large sample size
  • Stable process
  • Normal distribution

When these assumptions are not met, the values are not valid.

Most capability index estimates are valid only if the sample size used is “large enough,” which is generally thought to be about 30 or more independent data values.

Below, within the steps of a process capability analysis, we discuss how to determine stability and if a data set is normally distributed.

Steps for a Capability Analysis

To assure valid results when performing a capability analysis, follow these steps.

  1. Generate I and mR charts.
    • Start with the range chart and determine stability. Are all points inside of the control limits? If yes, the process is stable and the analysis can move forward. If no, the process is unstable and this must be addressed before moving on.
  2. Look at individuals chart.
    • Are there any indications of out-of-control data per the rules of process control? If yes, the process is out of control and this must be addressed before advancing in the capability analysis. If no, then the process is in control and analysis may proceed.
  3. Generate a normal probability chart and test for normality
    • If a distribution is close to normal, the normal probability plot will be close to a straight line. Minitab and other common software packages report the Anderson-Darling statistic. The null hypotheses for this test is that the distribution is normal; thus, to conclude that the data is normal, the p-value must be greater than 0.05 (typically).
    • If the data is normal, then assess capability. Otherwise the data may need to be transformed. A popular transformation is the Box-Cox transformation. (Alternatively, you could use a process capability index that applies to non-normal distributions. One statistic is called Cnpk [for non-parametric Cpk]. For additional information on non-normal distributions, see the book Process Capability Indices.)
  4. Assess process capability.
Figure 5: P-values
Figure 5: P-values

Resources

  1. Berardinelli, Carl. “A Guide to Control Charts,” iSixSigma.com. Accessed March 30, 2017. https://www.isixsigma.com/tools-templates/control-charts/a-guide-to-control-charts/.
  2. Berardinelli, Carl. “Multivariate Control Charts: T2 and Generalized Variance,” iSixSigma.com. Accessed March 30, 2017. https://www.isixsigma.com/tools-templates/control-charts/multivariate-control-charts-t2-and-generalized-variance/.
  3. Berardinelli, Carl. “Using Control Charts or Pre-control Charts,” iSixSigma.com. Accessed March 30, 2017. https://www.isixsigma.com/tools-templates/control-charts/using-control-charts-or-pre-control-charts/.
  4. Berardinelli, Carl and Yerian, Lew. “Run Charts: A Simple and Powerful Tool for Process Improvement,” iSixSigma.com. Accessed March 30, 2017. https://www.isixsigma.com/tools-templates/control-charts/run-charts-a-simple-and-powerful-tool-for-process-improvement/.
  5. Wheeler, Donald J. and Chambers, David S., Understanding Statistical Process Control (Tennessee: SPC Press, 1992).
  6. Quality Council of Indiana. The Certified Six Sigma Black Belt Primer, Second Edition (Indiana: Quality Council of Indiana, 2012).
  7. Tubiak, T.M. and Benbow, Donald W. The Certified Six Sigma Black Belt Handbook, Second Edition (Wisconsin: ASQ Quality Press, 2009).
  8. Pyzdek, Thomas. The Six Sigma Handbook (McGraw-Hill Education, 2003).
  9. Minitab Statistical Software V17.
  10. Krishnamoorthi, K.S., “Capability Indices for Processes Subject to Unilateral and Positional Tolerances,” Quality Engineering, Vol. 2, Issue 4, 461-471.
  11. Tahan, Antpine and Levesque, Sylvain. (2009). “Exploiting the process capability of position geometric tolerance according GD&T ASME Y14.5M,” doi: 10.1109/ICCIE.2009.5223885.
About the Author

Carl Berardinelli